Aku sedang membaca sesuatu di wikipedia dan membuat keputusan mungkin aku patut cuba memahami semua subjek matematik yang aku tak faham dulu. Mungkin dalam bahasa inggeris, subjek tu lebih mudah difahami sebab kadang2 BM ni terlalu bersifat menterjemah sampai tak paham.
Dan aku buat kesimpulan bahawa ia bukan salah cikgu, atau salah bahasa melayu.
Aku memang tak peduli. I just don't care. I just don't care if pi is the ratio of a circle's circumference to its diameter, or if it's ubiquitous in science and engineering. I just don't give a damn.
Geometric definition
In
Euclidean plane geometry, π is defined as the
ratio of a
circle's
circumference C to its
diameter d:
[9]
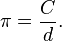
The ratio
C/
d is constant, regardless of a circle's size. For example, if a circle has twice the diameter
d of another circle it will also have twice the circumference
C, preserving the ratio
C/
d.
Alternatively π can be defined as the ratio of a circle's
area A to the area of a square whose side is equal to the
radius r of the circle:
[9][14]
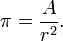
These definitions depend on results of Euclidean geometry, such as the fact that all circles are
similar, and the fact that the right-hand-sides of these two equations are equal to each other (i.e. the
area of a disk is
Cr/2). These two geometric definitions can be considered a problem when π occurs in areas of mathematics that otherwise do not involve geometry. For this reason, mathematicians often prefer to define π without reference to geometry, instead selecting one of its
analytic properties as a definition. A common choice is to define π as twice the smallest positive
x for which the
trigonometric function cos(
x) equals zero.
[15]
Circumference = π × diameter
Area of the circle equals π times the area of the shaded square
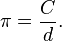
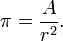


2 comments:
π = 3.142
hahaha..
been using 'pi' that often dah membuatkan aku terlupa the fundamental of 'pi' itself..thanks for reminding..eheh..
hahaha, reminder yg sendiri pon tak faham pi, never will and never want to. :P
Post a Comment